In my last post, I introduced the concepts of the efficient frontier and the capital allocation (or market) line and how it can be used to design portfolios that maximize return for a given risk level.
By utilizing these two concepts, and the mathematics behind them, portfolios made up of long positions in stocks, mutual funds, or ETFs can be designed for pretty much any risk level.
Understanding risk adjusted return
The concept of risk adjusted return can be used to compare the returns of portfolios with different risk levels against a benchmark with a known return and risk profile.
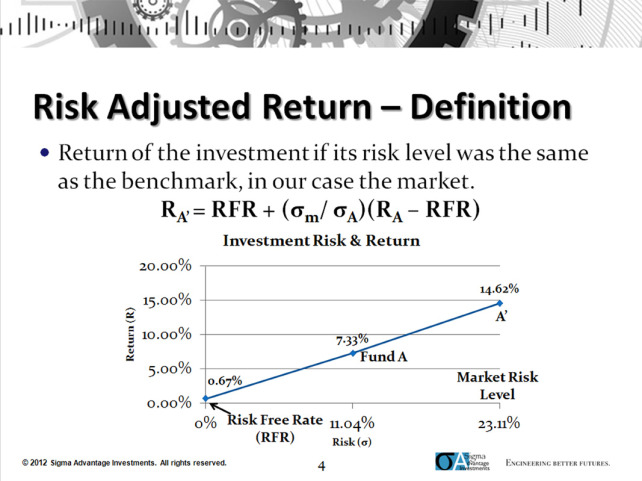
Figure 1 above shows the equation used to calculate the risk adjusted return for an investment.
The investment could be a mutual fund, stock, ETF, or a portfolio. The term (RA – RFR) subtracts the return of the risk free asset (e.g. U.S. Treasury Bills) from the return of the asset. This return is what is earned over and above the that earned risk free. This term is then multiplied by the ratio of the risk level of the market divided by the risk level of the asset (in this case the standard deviations of each).
If an asset has a lower risk level than the market, the return of the asset above the risk free rate is given a boost. If the asset has a higher than market risk level, the above risk free return is reduced.
Comparing market risk level
Our example in Figure 1, Fund A, has a lower than market risk level. Therefore, its above risk free return will obtain a boost of
(23.11% / 11.04%) = 2.0933.
We add the risk free rate back to the resulting above risk free rate to obtain the comparable return of the asset if it was at the same risk level of the benchmark, in this case the market.
RA’ = 0.67% + (23.11% / 11.04%)(7.33% – 0.67%)
RA’ = 0.67% + (2.0933)(6.66%)
RA’ = 14.62%
You may notice the line is similar to the capital allocation/market line, only this time the line is running from the risk free rate and through the risk and return of the asset, Fund A in this case. It is utilizing the same concept, but this time it allows use to calculate what the fund’s return would be if it was at the same risk level as the market.
It allows us to do a direct, or apples to apples, comparison between the return of the fund and the market while at the same time taking into consideration the difference in risk.
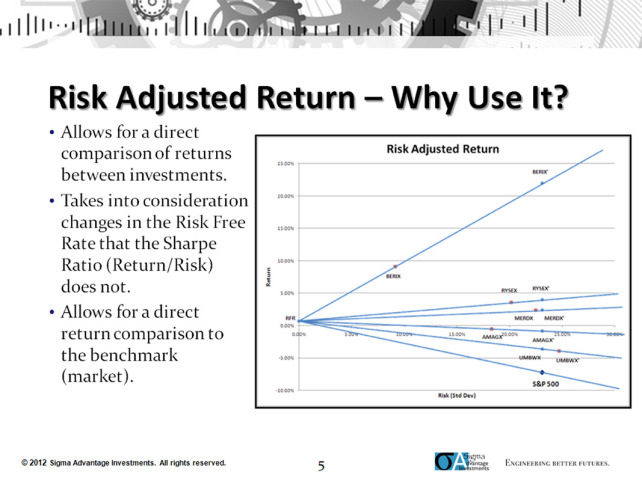
Why use risk adjusted return
Figure 2 below shows what this might look like for several investment options.
The line from the risk free rate through the S&P 500’s risk and return is the security market line, presented in this post.
